About Ken Stephenson
Welcome... I am a Professor Emeritus in the mathematics department at the University of Tennessee. My primary research interests revolve around circle packing: connections to analytic function theory, Riemann surfaces, computational conformal structures, and applications.

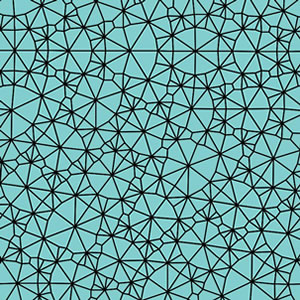
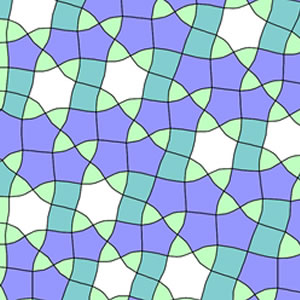
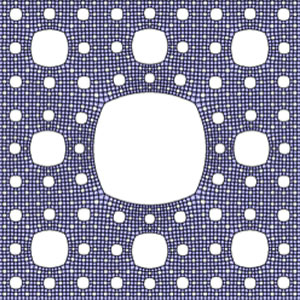
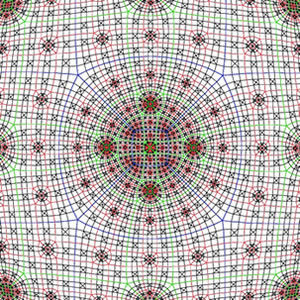
Circle Packing Research
A circle packing is a configuration of circles with a specified pattern of tangencies. The circles typically must assume a variety of different sizes in order to fit together in a prescribed tangency pattern. It is easy to confuse this with the well known topic of 'sphere packing', how many ping pong balls fit in a box car, but there is almost no contact between these two topics.

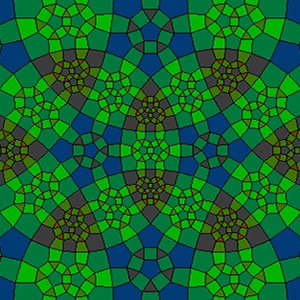
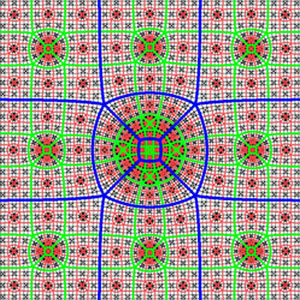
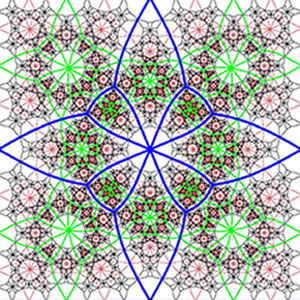
History of Circle Packing
Circle packings were first introduced by William Thurston in his Notes. Maps between circle packings, which preserve tangency and orientation, act many ways as discrete analogues of analytic functions. Moreover, work flowing from a 1985 conjecture of Thurston, proven by Burt Rodin and Dennis Sullivan, shows that classical analytic functions and more general classical conformal objects can be approximated using circle packings. Circle packings are computable, so they are introducing an experimental, and highly visual, component to research in conformal geometry and related areas. Circle packings are also useful in graph embedding, and have interesting connections to random walks. Below are images from the book I wrote, "Introduction to Circle Packing: The Theory of Discrete Analytic Functions", Cambridge University Press (2005).

Additional Topics I Enjoy
Analysis, computational and applied mathematics, and probability and stochastic processes. Feel free to contact me if you have questions about my research, or would like to discuss any other topics.